Sum of Angles in a Pentagon

Given square and rectangle. For a tessellation composed of polygons the sum of the angles formed at any vertex equals 360.

Image Result For Polygon Interior Angles Regular Polygon Math Charts Quadrilaterals

Perpendicular regression fits a line to data points by minimizing the sum of squared perpendicular distances from the data points to the line.

. Sum of angles at a vertex. Hence the sum of interior angles of a pentagon is 540. Its interior angles add up to 3 180 540 And when it is regular all angles the same then each angle is 540 5 108 Exercise.

In geometry a pentagon from the Greek πέντε pente meaning five and γωνία gonia meaning angle is any five-sided polygon or 5-gon. 360 div number of sides. A 5-sided polygon is called a pentagon for example.

The angles at a vertex to the right are 120120120360. A pentagon may be simple or self-intersectingA self-intersecting regular pentagon or star pentagon is called a pentagram. Difference of two angles.

The formula is where is the sum of the interior angles of the polygon and equals the number of sides in the polygon. Make sure each triangle here adds up to 180 and check that the pentagons interior angles. The formula for calculating the size of an exterior angle in a regular polygon is.

Many polygons have names based on the number of sides. The sum of the internal angles in a simple pentagon is 540. Set up the formula for finding the sum of the interior angles.

Hence each interior angle. Sum of the exterior angles of polygons 360 The sum will always be equal to 360 degrees irrespective of the number of sides it has. The largest pentagon that will fit in the circle with each vertex touching the circle.

There are some that wish to name every possible polygon but there seems little point in doing so. Here n is 5 as the pentagon has 5 sides. The sum of the exterior angles of a polygon is 360.

Since a pentagon has 5 sides the sum of interior angles of a pentagon is 5-2 180 where n5 3 180 540. The sum of interior angles of any polygon can be calculated using a formula. S n 2 180 Here n 10.

The value 180 comes from how many degrees are in a triangle. Given sum of angles. For the tessellation above composed of squares to the left the sum of the angles at a vertex are 90909090360.

Find the measure of each interior angle of a regular decagon. What is the Sum of all Interior Angles of a Pentagon. A decagon has ten sides.

The sum of all the interior angles of any pentagon is always equal to 540. There are also angles on a straight line worksheets based on Edexcel AQA and OCR exam questions along with further guidance on where to go next if. Thus the sum of the interior.

Angles on a Straight Line. Now to find the measure of the interior angles of the pentagon we know that the sum of all the angles in a pentagon is equal to 540 degrees from the above figureand there are five angles. The other part of the formula is a way to determine how many triangles the polygon can be divided into.

Each exterior angle must be 360n. Here we will learn about angles on a straight line including the sum of angles on a straight line how to find missing angles and using these angle facts to generate equations and solve problems. The distance from a point to a plane is measured as the length from the point along a segment that is perpendicular to the plane meaning that it is perpendicular to all lines in the plane that pass through the nearest point in.

In a regular polygon all its. Since the interior angles of a regular pentagon are equal we have to divide 540 by 5 to find the measure of each interior angle. Therefore by the angle sum formula we know.

Sum of n angles. Therefore the sum of angles in a pentagon is 540 degrees. First of all we can work out angles.

The formula is derived considering that we can divide any polygon into triangles. Here m n o p q 360 Angles in Regular Polygon. Also a regular pentagon has all its interior angles with the same measure.

Sum of the exterior angles of polygons. Hence sum is 180n-2 1805-2 180 3 540. An Interior Angle is an angle inside a shape.

The Exterior Angle is the angle between any side of a shape and a line extended from the next side. Lets calculate the sum of the interior angles of a pentagon using the sum of interior angles formula S 180n-2 where n is the number of sides in a polygon. We know that the formula to calculate the sum of interior angles of a polygon is n 2 180.

For example a 42-sided polygon is called a tetracontakaidigon. Hence Sum of angles of pentagon 10 2 180 S 8 180 S 1440. A pentagon has 5 sides and can be made from three triangles so you know what.

If the polygon is regular we can calculate the measure of one of its interior angles by dividing the total sum by the number of sides of the polygon. Prove equal angles equal sides and altitude. Sum of angles of pentagon 5 2 180 S 3 180 S 540 Question 2.

Sum of Exterior Angles in a Pentagon. Learn more about Pentagon Regular and Irregular Pentagons and their shape with Formulas Definitions Examples Properties Area Perimeter etc. Constructing 75 105 120 135 150 angles and more.

All the Exterior Angles of a polygon add up to 360 so. Beyond about 10 sides most people call them an n-gon. In geometry a pentagon is a five-sided polygon with five straight sides and five interior angles which add up to 540.

How to construct draw a regular pentagon inscribed in a circle. Consider the following polygon with 5 sides. 3 x 180 540 degrees.

Since the sum of the angles of the triangles is equal to 180 degrees. Given square area and triangle area.

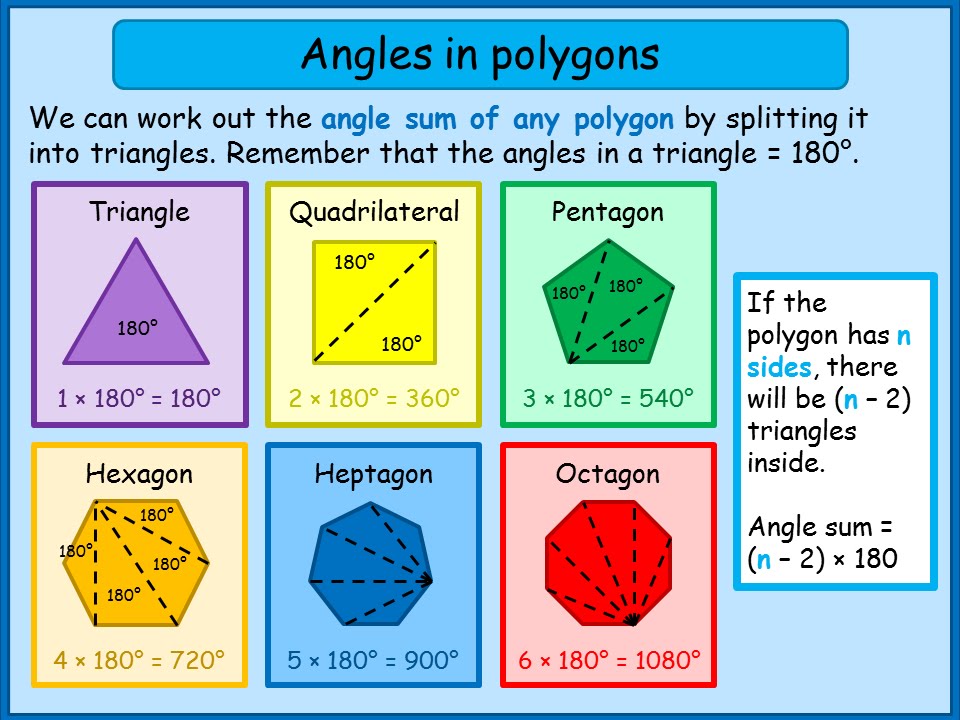
11 Angle Sum Of Any Polygon Maths Tutorials Youtube Math Tutorials Math For Kids Math Activities

11 Angle Sum Of Any Polygon Maths Tutorials Youtube Math Tutorials Math For Kids Math Activities

Polygons Polygon Quadrilaterals Geometry Formulas

Polygon Worksheets Sum Of Interior Angles Of Polygons Worksheet Angles Worksheet Regular Polygon Word Problem Worksheets